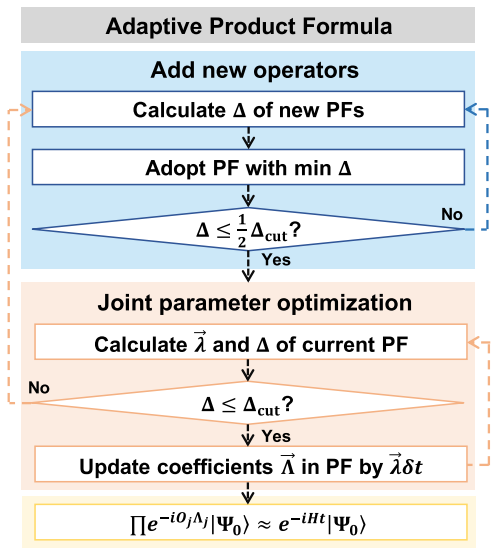
Various Hamiltonian simulation algorithms have been proposed to efficiently study the dynamics of quantum systems on a quantum computer. The existing algorithms generally approximate the time evolution operators, which may need a deep quantum circuit that is beyond the capability of near-term noisy quantum devices. Here, focusing on the time evolution of a fixed input quantum state, we propose an adaptive approach to construct a low-depth time evolution circuit. By introducing a measurable quantifier that characterizes the simulation error, we use an adaptive strategy to learn the shallow quantum circuit that minimizes that error. We numerically test the adaptive method with electronic Hamiltonians of the H2O and H4 molecules, and the transverse field Ising model with random coefficients. Compared to the first-order Suzuki-Trotter product formula, our method can significantly reduce the circuit depth (specifically the number of two-qubit gates) by around two orders while maintaining the simulation accuracy. We show applications of the method in simulating many-body dynamics and solving energy spectra with the quantum Krylov algorithm. Our work sheds light on practical Hamiltonian simulation with noisy-intermediate-scale-quantum devices.

Paper: https://journals.aps.org/prl/abstract/10.1103/PhysRevLett.130.040601